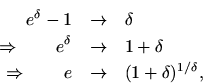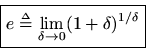Back to e

01 Mar 1998

NOTE: THIS DOCUMENT IS OBSOLETE, PLEASE CHECK THE NEW VERSION: "Mathematics of the Discrete Fourier Transform (DFT), with Audio Applications --- Second Edition", by Julius O. Smith III, W3K Publishing, 2007, ISBN 978-0-9745607-4-8. - Copyright © 2017-09-28 by Julius O. Smith III - Center for Computer Research in Music and Acoustics (CCRMA), Stanford University

<< Previous page TOC INDEX Next page >>

Back to e

Above, we defined as the particular real number satisfying

which gave us $$(a^x)^\$ when . From this expression, we have, as $$\$ ,

or,

This is one way to define . Another way to arrive at the same definition is to ask what logarithmic base gives that the derivative of $$\$ is . We denote $$\$ by $$\$ .
<< Previous page TOC INDEX Next page >>

GUIDE: Mathematics of the Discrete Fourier Transform (DFT) - Julius O. Smith III. Back to e

Back to e