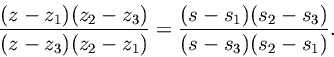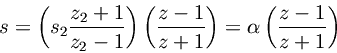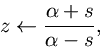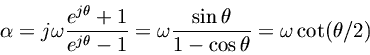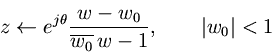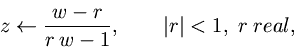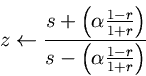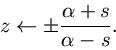04. Relation to functions positive real in the right-half plane

01 Mar 1998

NOTE: THIS DOCUMENT IS OBSOLETE, PLEASE CHECK THE NEW VERSION: "Introduction to Digital Filters with Audio Applications", by Julius O. Smith III, Copyright © 2017-11-26 by Julius O. Smith III - Center for Computer Research in Music and Acoustics (CCRMA), Stanford University

<< Previous page TOC INDEX Next page >>

Relation to functions positive real in the right-half plane

Theorem. $$\$ , where $$\$ is any positive real number.

Proof. We shall show that the change of variable $$z\$ , provides a conformal map from the z-plane to the s-plane that takes the region $$\$ to the region $$\$ . The general formula for a bilinear conformal mapping of functions of a complex variable is given by

In general, a bilinear transformation maps circles and lines into circles and lines [Churchill 1960]. We see that the choice of three specific points and their images determines the mapping for all and . We must have that the imaginary axis in the s-plane maps to the unit circle in the z-plane. That is, we may determine the mapping by three points of the form $$z_i=e^{j\$ and $$s_i=j\$ . If we predispose one such mapping by choosing the pairs $$(s_1=\$ and $$(s_3=0)\$ , then we are left with transformations of the form

or

Letting be some point $$j\$ on the imaginary axis, and be some point $$e^{j\$ on the unit circle, we find that

which gives us that $$\$ is real. To avoid degeneracy, we require $$s_2\$ , and this translates to $$\$ finite and nonzero. Finally, to make the unit disk map to the left-half s-plane, $$\$ and $$\$ must have the same sign in which case $$\$ . $$\$
There is a bonus associated with the restriction that $$\$ be real which is that

We have therefore proven

Theorem. PR $$ \$ PR, where $$\$ is any positive real number.

The class of mappings of the form Eq. (1.3) which take the exterior of the unit circle to the right-half plane is larger than the class Eq. (1.3). For example, we may precede the transformation Eq. (1.3) by any conformal map which takes the unit disk to the unit disk, and these mappings have the algebraic form of a first order complex allpass whose zero lies inside the unit circle.

where is the zero of the allpass and the image (also pre-image) of the origin, and $$\$ is an angle of pure rotation. Note that Eq. (1.3) is equivalent to a pure rotation, followed by a realallpass substitution ( real), followed by a pure rotation. The general preservation of condition (2) in Def. 2 forces the real axis to map to the real axis. Thus rotations by other than $$\$ are useless, except perhaps in some special cases. However, we may precede Eq. (1.3) by the first order real allpass substitution

which maps the real axis to the real axis. This leads only to the composite transformation,

which is of the form Eq. (1.3) up to a minus sign (rotation by $$\$ ). By inspection of Eq. (1.3), it is clear that sign negation corresponds to the swapping of points and , or and . Thus the only extension we have found by means of the general disk to disk pre-transform, is the ability to interchange two of the three points already tried. Consequently, we conclude that the largest class of bilinear transforms which convert functions positive real in the outer disk to functions positive real in the right-half plane is characterized by

Riemann's theorem may be used to show that Eq. (1.3) is also the largest such class of conformal mappings. It is not essential, however, to restrict attention solely to conformal maps. The pre-transform $$z\$ , for example, is not conformal and yet PR is preserved.
The bilinear transform is one which is used to map analog filters intodigital filters. Another such mapping is called the matched transform [Rabiner and Gold 1975]. It also preserves the positive real property.

Theorem. is PR if $H(e^{sT})$ is positive real in the analog sense, where is interpreted as the sampling period.

Proof. The mapping $$z \$ takes the right-half -plane to the outer disk in the -plane. Also is real if is real. Hence $H(e^{sT})$ PR implies PR. (Note, however, that rational functions do not in general map to rational functions.) $$\$

These transformations allow application of the large battery of tests which exist for functions positive real in the right-half plane [Van Valkenburg 1960].