Orthogonality of Sinusoids

GUIDE: Mathematics of the Discrete Fourier Transform (DFT) - Julius O. Smith III. Orthogonality of Sinusoids

It appears that you are using AdBlocking software. The cost of running this website is covered by advertisements. If you like it please feel free to a small amount of money to secure the future of this website.
​

NOTE: THIS DOCUMENT IS OBSOLETE, PLEASE CHECK THE NEW VERSION: "Mathematics of the Discrete Fourier Transform (DFT), with Audio Applications --- Second Edition", by Julius O. Smith III, W3K Publishing, 2007, ISBN 978-0-9745607-4-8. - Copyright © 2017-09-28 by Julius O. Smith III - Center for Computer Research in Music and Acoustics (CCRMA), Stanford University

<< Previous page  TOC  INDEX  Next page >>

Orthogonality of Sinusoids

A key property of sinusoids is that they are orthogonal at different frequencies. That is,

\

This is true whether they are complex or real, and whatever amplitude and phase they may have. All that matters is that the frequencies be different. Note, however, that the sinusoidal durations must be infinity.

For length $N$ sampled sinuoidal signal segments, such as used by the DFT, exact orthogonality holds only for the harmonics of the sampling rate divided by $N$, i.e., only over the frequencies $f_k = k f_s / N, k=0,1,2,3,\. These are the only frequencies that have an exact integer number of periods in $N$samples (depicted in Fig. 7.2 for $N=8$).

The complex sinusoids corresponding to the frequencies $f_k$ are

\

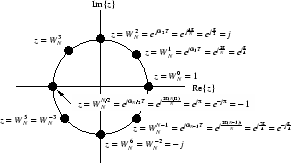
These sinusoids are generated by the $N$ roots of unity in the complex plane:
\

These are called the $N$ roots of unity because each of them satisfies
\

The $N$ roots of unity are plotted in the complex plane in Fig. 7.1for $N=8$. In general, for any $N$, there will always be a point at $z=1$, and the points equally subdivide the unit circle. When $N$ is even, there is a point at $z=-1$ (corresponding to a sinusoid at exactly half the sampling rate), while if $N$ is odd, there is no point at $z=-1$.
Figure 7.1:The $N$ roots of unity for $N=8$.
\

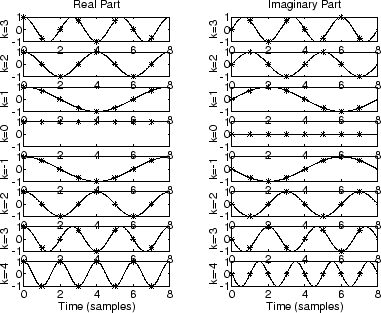
The sampled sinusoids corresponding to the $N$ roots of unity are plotted in Fig. 7.2. These are the sampled sinusoids $(W_N^k)^n = e^{j 2 \ used by the DFT. Note that taking successively higher integer powers of the point $W_N^k$ on the unit circlegenerates samples of the $k$th DFT sinusoid, giving $[W_N^k]^n$, $n=0,1,2,\. The $k$th sinusoid generator $W_N^k$ is in turn the$k$th power of the primitive $N$th root of unity $W_N \. The notation $W_N$, $W_N^k$, and $W_N^{nk}$ are common in the digital signal processing literature.

Figure 7.2:The $N$ complex sinusoids used by the DFT for $N=8$.
\

Note that in Fig. 7.2 the range of $k$ is taken to be $[-N/2,N/2-1] = [-4,3]$ instead of $[0,N-1]=[0,7]$. This is the most ''physical'' choice since it corresponds with our notion of ''negative frequencies.'' However, we may add any integer multiple of $N$ to $k$without changing the sinusoid indexed by $k$. In other words, $k\ refers to the same sinusoid for all integer $m$.

<< Previous page  TOC  INDEX  Next page >>

​
 
​
© 1998-2023 – Nicola Asuni - Tecnick.com - All rights reserved.
about - disclaimer - privacy